Solution for f (x)=a (xh)2k equation Simplifying f (x) = a (x 1h) * 2 k Multiply f * x fx = a (x 1h) * 2 k Reorder the terms fx = a (1h x) * 2 k Reorder the terms for easier multiplication fx = 2a (1h x) k fx = (1h * 2a x * 2a) k fx = (2ah 2ax) k Solving fx =2 x 1 = f(x 2) f(x 1) x 2 x 1 (61) It's a linear approximation of the behavior of f between the points x 1 and x 2 7 Quadratic Functions The quadratic function (aka the parabola function or the square function) f(x) = ax2 bx c (71) can always be written in the form f(x) = a(x h)2 k (72) where V = (h;k) is the coordinate of the vertexA quadratic function is a polynomial function of degree two The graph of a quadratic function is a parabola The general form of a quadratic function is f(x) = ax2 bx c where a, b, and c are real numbers and a ≠ 0 The standard form of a quadratic function is f(x) = a(x − h)2 k where a ≠ 0

Quadratic Functions
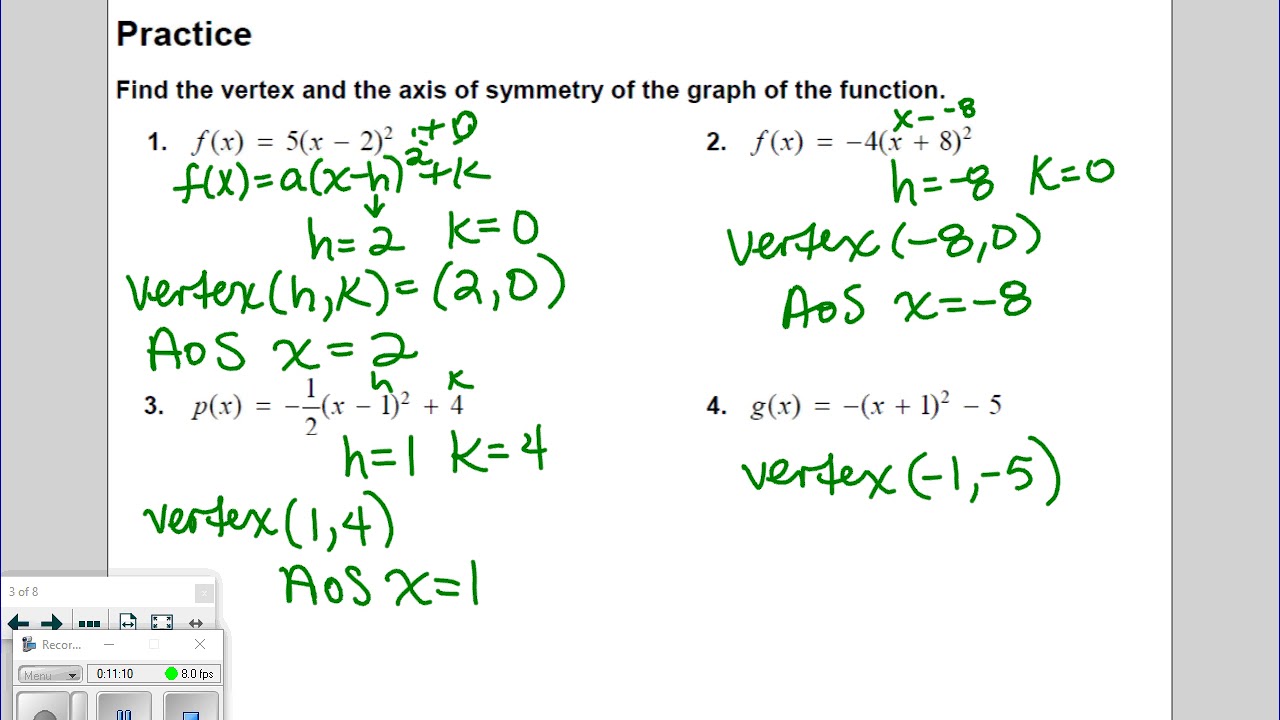
F(x)=a(x-h)^2+k
F(x)=a(x-h)^2+k-Write the function in the form $f(x)=a(xh)^{2}k$ by completing the square Then identify the vertex $$q(x)=2 x^{2}12 x11$$Spanish2B Chapter 46 Vocabulary Words 214 terms Claire_Marie8 x=a (yk)^2h 7 terms Claire_Marie8 1450present 35 terms Claire_Marie8




Pre Calculus 2 6a Page 160 S 1 23odd Ppt Video Online Download
Substitute ah and a for x in the formula for f(x) and simplify to find (f(ah)f(a))/h = 2a 2 h >f(x) = x^22x3 Then (f(ah) f(a))/h =(((ah)^22(ah)366E 67E The quadratic function f ( x) = a ( x − h)2 k is in standard form ( a) The graph of f is a parabola with vertex (____, ____) (b) If a > 0, the graph of f opens ________ In this case f ( h) = k is the _______ value of f (c) If a < 0, the graph of f opens f(x) = a(x − h)2 k form Determine the vertex and the axis of symmetry of the graph of the function f(x) = x2 4x − 5
This video shows how to use horizontal and vertical shifts together to graph a radical functionFor the base function f (x) and a constant k > 0, the function given by g(x) = k f (x), can be sketched by vertically stretching f (x) by a factor of k if k > 1 or by vertically shrinking f (x) by a factor of k if 0 < k < 1 Horizontal Stretches and Shrinks For the base function f (x) and a constant k, where k > 0 and k ≠ 1, theQuestion Express f ( x) in the form a ( x − h) 2 k f (x) = − 3/4x 2 15x − 77
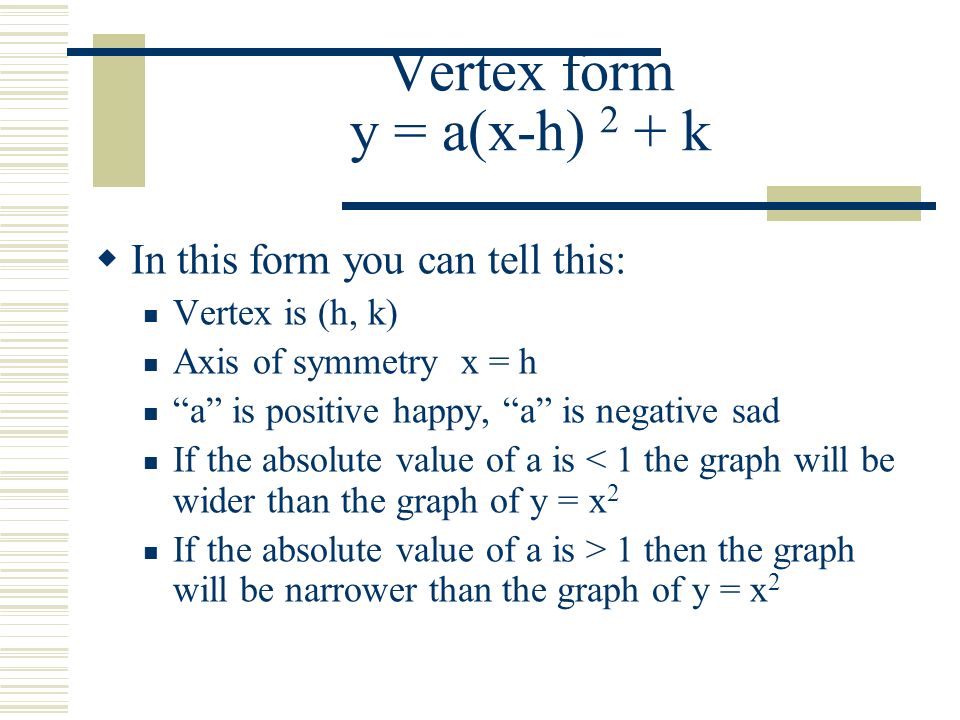
ProofLet fK g 2A be a family of convex sets, and let K = \ 2AK Then, for any x;y2 K by de nition of the intersection of a family of sets, x;y2 K for all 2 nd each of these sets is convex Hence for any 2 A;and 2 0;1;(1 )x y2 KConsider the graph of the parabola y=ax^2 Its vertex is clearly at (0,0) Now, if you replace x with xh in any equation, its graph gets shifted to the right by a distance of hGraphing f (x) = a(x − h)2 k The vertex form of a quadratic function is f (x) = a(x − h)2 k, where a ≠ 0 The graph of f (x) = a(x − h)2 k is a translation h units horizontally and k units vertically of the graph of f (x) = ax2 The vertex of the graph of f (x) = a(x − h)2 k is (h, k), and the axis of symmetry is x = h h f(x) = ax2 y x f(x) = a(x − 2h) k k (h, k)



Why Is It In Vertex Form Of Quadratic Function Y A X H 2 K Getting Value Of H Is Opposite To Its Value Quora



Solved Use The Graph Of The Quadratic Function F X A X H 2 K To Find The Vertex Axis Of Symmetry And The Minimum Or Maximum Value Of The Functi Course Hero
F(x) = a(x h) 2 k The a in the vertex form of a parabola corresponds to the a in standard form If a is positive, the parabola will open upwards If a is negative, the parabola will open downwards In vertex form, (h,k) describes the vertex of the parabola and the parabola has a line of symmetry x = hThe standard form of a quadratic function presents the function in the form latexf\left(x\right)=a{\left(xh\right)}^{2}k/latex where latex\left(h,\text{ }k\right)/latex is the vertex Because the vertex appears in the standard form of the quadratic function, this form is also known as the vertex form of a quadratic function The standard form is useful for determiningFor instance, when D is applied to the square function, x ↦ x 2, D outputs the doubling function x ↦ 2x, which we named f(x) This output function can then be evaluated to get f(1) = 2, f(2) = 4, and so on Higher derivatives Let f be a differentiable function, and let f ′ be its derivative



Review Melody Davis And Jessica Perry




Quadratic Functions
For a function f (x), the difference quotient would be f(xh) f(x) / h, where h is the point difference and f(xh) f(x) is the function difference The difference quotient formula helps to determine the slope for the curved lines The f(xh) f(x) / h calculator can be used to find the slope value, when working with curved linesA polynomial function in one variable of degree 2 Polynomial form f(x)= a 2 x 2 a 1 x a 0 Standard form 1 f(x) = ax 2 bx c Standard form 2 f(x) = a (xh) 2 k Cubic function A polynomial function in one variable of degree 3 Polynomial form f(x)= a 3 x 3 a 2 x 2 a 1 x a 0 Quartic function A polynomial function in oneX k ∆kf(a) k!



8 4 Graphing F X A X H 2 K Youtube



Section 5 3 Transforming Parabolas Standard Form Vs Vertex Form Standard Form Is Y Ax 2 Bx C Vertex Form Is Y A X H 2 K Ppt Download
Explore the parent graph y=x^3 Experiment with the values of a, h, and k What happens to the graph as these values change?I will answer this way, since I suspect you're preparing for finals The x and y are the variables They aren't replaced with specific numbers in the equation unless you are finding (x, y) coordinates for points on the parabola The h is a horizonThe submatrix H i, j is of dimension P 2 × P 2 and represents the contribution of the jth band of the input to the ith band of the output Since an optical system does not modify the frequency of an optical signal, H will be block diagonal There are cases, eg, imaging using color filter arrays, where the diagonal assumption does not hold




Write The Quadratic Function In F X A X H 2 K Form Whose Graph Is Shown F X Brainly Com



Sections 6 And 8 Quadratics Part 2 And Polynomial Functions
#h'(x)=f'(x)g(x)f(x)g'(x)# We are ask to find #h'(1)#, or by the product rule #h'(1)=f'(1)g(1)f(1)g'(1)# The values of the functions must be #f(1)=2# and #g(1)=4/3# Remember the derivative gives the slope of any given point, but as we can see in the figures these must correspond, to the slope of the line, which goes through theF (x) = a*log (xh)k f (x) = a*2^ (xh) k i think the k is the horizontal asymptope butSee the answer The quadratic function f (x) = a (x − h)2 k is in standard form (a) The graph of f is a parabola with vertex (x, y) = (b) If a > 0, the graph of f opens In this case f (h) = k is the value of f (c) If a < 0, the graph of f opens In this case f (h) = k




The Graph Of F X X 2 Has Been Shifted Into The Form F X X H 2 K What Is The Value Of H A 1 B Brainly Com




Quadratic Functions



0 件のコメント:
コメントを投稿