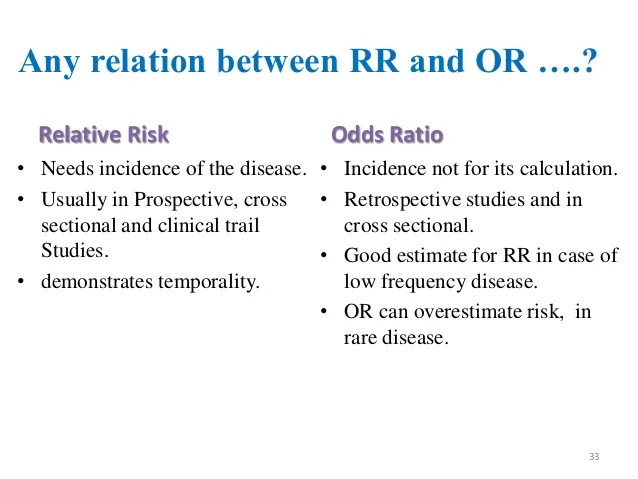
Expected odds ratio This is what you expect the odds ratio to be, ie, the odds of the outcome given presence of the property you are looking for an association with relative to the same outcome in the absence of that property This can often be determined by using the results from a previous study, or by running a small pilot studyEnglishwise, they are correct it is the odds and the odds are based on a ratio calculation It is not , however, the odds ratio that is talked about when results are reported The odds ratio when results are reported refers to the ratio of two odds or, if you prefer, the ratio of two odds ratios That is, let us writeLet us now look at the relation between the relative risk and the odds ratio (Zhang and Yu, 1998) OR= ˇ 1 1 1ˇ 1 ˇ 2 1 ˇ 2 = ˇ ˇ 2 1 2 1 1 = RR 2 1 (21) From this we see that OR is always further away from 1 than RR But, more importantly, we see that the odds ratio is close to the relative risk if probabilities of the outcome are small (Davies et al, 1998)

The Diagnostic Odds Ratio A Single Indicator Of Test Performance Journal Of Clinical Epidemiology
What is the difference between odds ratio and hazard ratio
What is the difference between odds ratio and hazard ratio-And a risk of 095 is equivalent to odds of 19 Measures of effect for clinical trials with dichotomous outcomes involve comparing either risks or odds from two intervention groups To compare them we can look at their ratio (risk ratio or odds ratio) or their difference in risk (riskA value lower than 100 indicates decreased risk The 95% confidence intervals and statistical




Bravură Fii Entuziasmat Analitic Odds Ratio Calculator Callumluckwellfinalyear Com
Risk ratios At a minimum, the only change that needs to be done to get risk ratios is to change the link function that relates the mean value of the response variable to the linear predictor For estimates of odds ratios, this is logit (ie the logarithm of the odds of the mean);After converting the odds ratio to a risk ratio, the actual risk is 14 (mortality is 14 times more likely in patients with ICU delirium compared to those without ICU delirium) Because the incidence rate in the nondelirium group is high, the odds ratio exaggerates the true risk demonstrated inTo the Editor Dr Norton and colleagues 1 described significant limitations of odds ratios (ORs) but they did not report one important advantage of ORs compared with risk ratios (RRs) the magnitude of the association between an exposure and a dichotomous outcome is invariant to whether the outcome is defined as event occurrence (eg, death) or nonoccurrence (eg, no death;
In prospective trials, it is simply a different way of expressing this association than relative riskTo calculate the odds ratio, we use one of the following formulas (both give the same outcome) Example 2 We compare smokers and nonsmokers with regard to the presence of ischemic heart disease The following table shows the results Example 2 We can now calculate the odds ratio (10/40) / (5/45) = 225More on the Odds Ratio Ranges from 0 to infinity Tends to be skewed (ie not symmetric) "protective" odds ratios range from 0 to 1 "increased risk" odds ratios range from 1 to Example "Women are at 144 times the risk/chance of men" "Men are at 069 times the risk
The ratio of two cumulative incidences is called the cumulative incidence risk ratio An odds ratio is the ratio of two odds for example a/b / c/d In epidemiological parlance it is the odds of infection for those exposed to a risk factor, divided by the odds of infection for those not exposed to that risk factorRelative Risk and Odds Ratio for the obese 3) Overall, you can see that decreasing the baseline incidence will decrease the odds ratio (300 in those who are nonobese versus 129 in those who are obese) Obviously, these results run counter to expected results, putting the onus on the researcher to justify them Similarly, you should find that increasing the incidence will increase the odds ratio Note that an odds ratio is a good estimate of the risk ratio when the outcome occurs relatively infrequently (




Odds Ratios The Odd One Out Stats By Slough




What Is An Odds Ratio And How Do I Interpret It Critical Appraisal
If the outcome is rare in both exposed and unexposed persons, the odds ratio (A/B/C/D) will approximate the risk ratio (A/(A B)/C/(C D)) However, when the study outcome is common and the risk ratio is not close to 1, the odds ratio will be further from 1 compared with the risk ratio9222 Measures of relative effect the risk ratio and odds ratio Measures of relative effect express the outcome in one group relative to that in the other The risk ratio (or relative risk) is the ratio of the risk of an event in the two groups, whereas the odds ratio is the ratio of the odds of an event (see Box 92a) For both measures a value of 1 indicates that the estimated effects are theThe more common the disease, the larger is the gap between odds ratio and relative risk In our example above, p wine and p no_wine were 0009 and 0012 respectively, so the odds ratio was a good approximation of the relative risk OR = 0752 and RR = 075 If the risks were 08 and 09, the odds ratio and relative risk will be 2 very different numbers OR = 044 and RR = 0 Relative risk vs Odds ratio




The Diagnostic Odds Ratio A Single Indicator Of Test Performance Journal Of Clinical Epidemiology



Q Tbn And9gcs7g3 Oy3gxo7fbk7uvklwexnnbqcmd7m5bqd Ghq64ww9hd4dh Usqp Cau
Overview The term measures of effect includes ratios, differences, relative risks (RR), odds ratios Population attributable risk and population attributable risk factor are also measures of effect but more specifically are measures of population impact Ratios and Differences Risks (defined as the probability of a new occurrence disease among individuals in an initially diseaseAs an extreme example of the difference between risk ratio and odds ratio, if action A carries a risk of a negative outcome of 999% while action B has a risk of 990% the relative risk is approximately 1 while the odds ratio between A and B is 10 (1% = 01% x 10), more than 10 times higher Definition The Odds Ratio is a measure of association which compares the odds of disease of those exposed to the odds of disease those unexposed Formulae OR = (odds of disease in exposed) / (odds of disease in the nonexposed) Example I often think food poisoning is a good scenario to consider when interpretting ORs Imagine a group of friends went out to



Relative Risk Vs Odds Ratio Authorstream



44 45 46 47 48 49 50 51 52 53 54 55 Review 1 Similar To The Risk Ratio Here Are The Guidelines For Calculating A Confidence Interval For 2 Independent Samples And The Odds Ratio As The Parameter Of Interest 2 Note That Calculation Of The Confidence
Odds ratios (OR) are commonly reported in the medical literature as the measure of association between exposure and outcome However, it is relative risk that people more intuitively understand as a measure of association Relative risk can be directly determined in a cohort study by calculating a risk ratio (RR) Percent increase = (Risk Ratio lower bound – 1) x 100 Percent decrease = (1 – Risk Ratio upper bound) x 100 It's worth stating again when comparing two proportions close to 1 or 0, the risk ratio is usually a better summary than the raw difference Odds Ratios We now turn to odds ratios as yet another way to summarize a 2 x 2 table The ratio of these two probabilities R1/R2 is the relative risk or risk ratio Pretty intuitive If the program worked, the relative risk should be smaller than one, since the risk of failing should be smaller in the tutored group If the relative risk is 1, the tutoring made no difference at all



Measuring Of Risk



Relative Risk Ratios And Odds Ratios
In a control group The odds ratio (OR) is the odds of an event in an experimental group relative to that in a control group An RR or OR of 100 indicates that the risk is comparable in the two groups A value greater than 100 indicates increased risk;Diagnostic odds ratio In medical testing with binary classification, the diagnostic odds ratio ( DOR) is a measure of the effectiveness of a diagnostic test It is defined as the ratio of the odds of the test being positive if the subject has a disease relative to the odds of the test being positive if the subject does not have the disease Examples of measures of association include risk ratio (relative risk), rate ratio, odds ratio, and proportionate mortality ratio Risk ratio Definition of risk ratio A risk ratio (RR), also called relative risk, compares the risk of a health event (disease, injury, risk factor, or death) among one group with the risk among another group
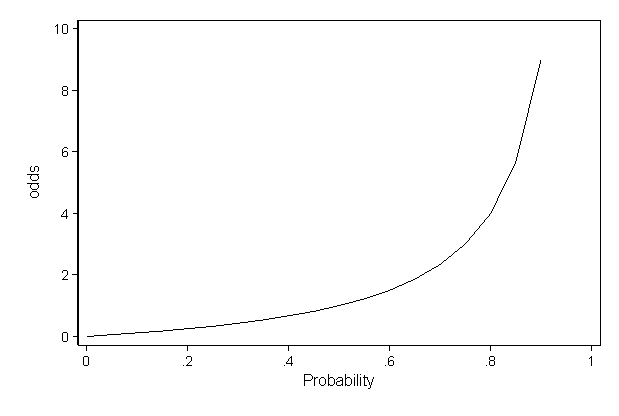



Faq How Do I Interpret Odds Ratios In Logistic Regression
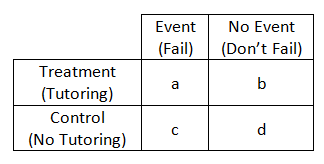



The Difference Between Relative Risk And Odds Ratios The Analysis Factor



0 件のコメント:
コメントを投稿